传送门
题意: 给出 n 个数排成一排,要求你对其中的数进行增加,使得每个数占其左侧所有数(前缀和,不包括它本身)的 k% 以下。求得最小的修改数量。
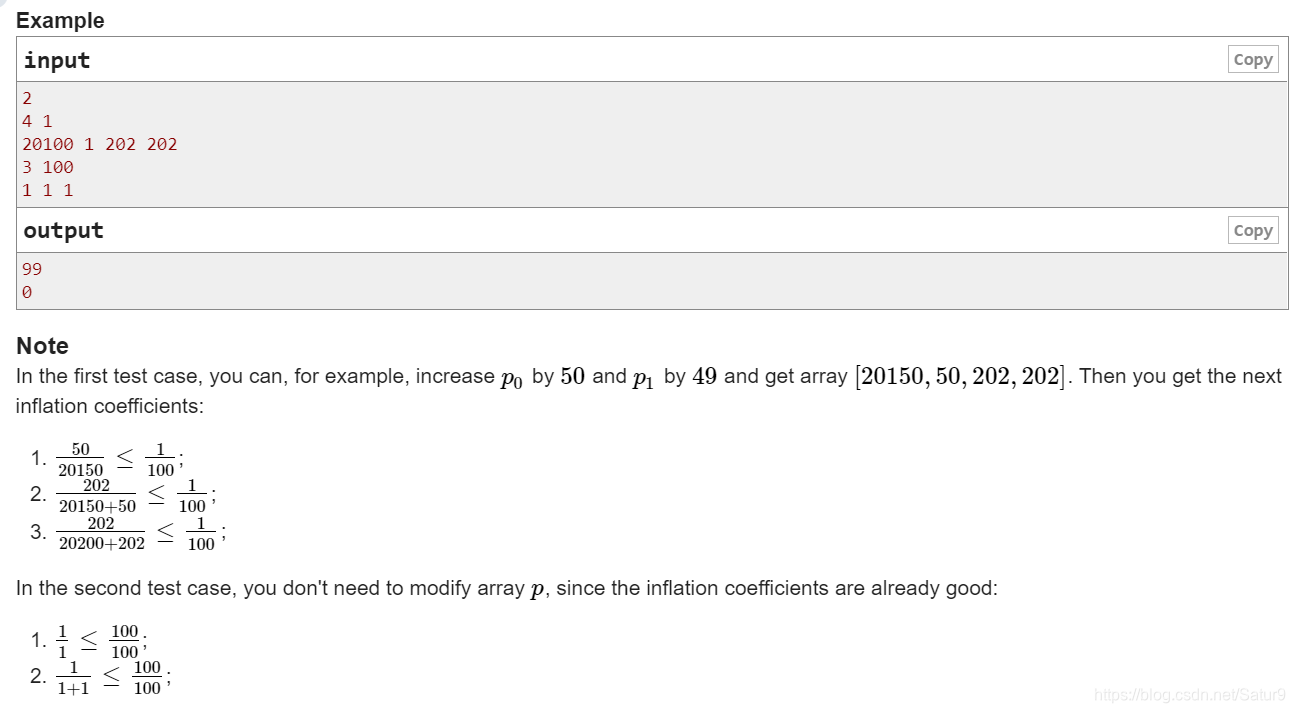
思路:
- 既然是前缀和的占比,并且第一个是 a[1] 没有前缀和,那么我们把所有增加量都放在 a[1] 即可。
- 从左往右遍历,找到不符合条件的数,并用其增加量来更新答案取最大值就好。
代码实现:
#include<bits/stdc++.h>
#define endl '\n'
#define null NULL
#define ll long long
#define int long long
#define pii pair<int, int>
#define lowbit(x) (x &(-x))
#define ls(x) x<<1
#define rs(x) (x<<1+1)
#define me(ar) memset(ar, 0, sizeof ar)
#define mem(ar,num) memset(ar, num, sizeof ar)
#define rp(i, n) for(int i = 0, i < n; i ++)
#define rep(i, a, n) for(int i = a; i <= n; i ++)
#define pre(i, n, a) for(int i = n; i >= a; i --)
#define IOS ios::sync_with_stdio(0); cin.tie(0);cout.tie(0);
const int way[4][2] = {
{
1, 0}, {
-1, 0}, {
0, 1}, {
0, -1}};
using namespace std;
const int inf = 0x7fffffff;
const double PI = acos(-1.0);
const double eps = 1e-6;
const ll mod = 1e9 + 7;
const int N = 2e5 + 5;int t, n, k;
int a[N], pre[N];signed main(){
IOS;cin >> t;while(t --){
cin >> n >> k;for(int i = 1; i <= n; i ++){
cin >> a[i];pre[i] = a[i]+pre[i-1];}int ans = 0;for(int i = 2; i <= n; i ++){
if(pre[i-1]*k<100*a[i])ans = max(ans,100*a[i]-pre[i-1]*k);}cout << ans/k+(ans%k ? 1:0) << endl;}return 0;
}